
So, on this page, we will cover all the equations for a falling body along with the free fall physics formula, and then derive the free fall formula as well. As there is a rate of change of velocity in an object while free-falling, so we can determine the free-fall acceleration formula as well. The eight can be calculated by using the maximum height formula free fall. Since the object falling from a mountain has a maximum height, so does the object. An object possessing a free-fall object formula bears velocity, which we can calculate by using the free-fall velocity formula. As it covers a certain distance, we can describe the free fall distance formula. Now, because of gravity (g), the body falls in the following manner:įrom this example, we can describe the free-fall motion formula. A free-fall concept that talks about the body freely falling under gravity.Īssume that a body with velocity v is descending freely from a mountain of height (h) for time (t) seconds. How high will it go before it begins to fall (g = 9.A free-falling object formula describes the self-governing phenomena of the body having some mass. Question A ball is thrown up with a speed of 15 m/s. Speed with which ball hits ground is 20 m/sįinding the time it takes to fall through this height (Acceleration is negative because ball is thrown upwards)Ī cricket ball is dropped from a height of 20 meters.Ĭalculate the speed of the ball when it hits the ground.Ĭalculate the time it takes to fall through this height (g = 10 m/s2)įinding speed of the ball when it hits the ground. (Acceleration is positive because stone falls downwards) Height of bridge will be the distance travelled by stone Calculate the height of the bridge from the water level (g = 9.8 m/s2) The stone takes 2 Seconds to touch the water surface in the river. To estimate the height of a bridge over a river, a stone is dropped freely in the river from the bridge. Find (a) the velocity with which it was thrown up, (b) the maximum height it reaches, and (c) its position after 4 s. Calculate when and where the two stones will meet.Ī ball thrown up vertically returns to the thrower after 6 s.

What is the net displacement & total distance covered by the stone?Ī stone is allowed to fall from the top of a tower 100 m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25 m/s. Taking g = 10 m/s2, find the maximum height reached by the stone.
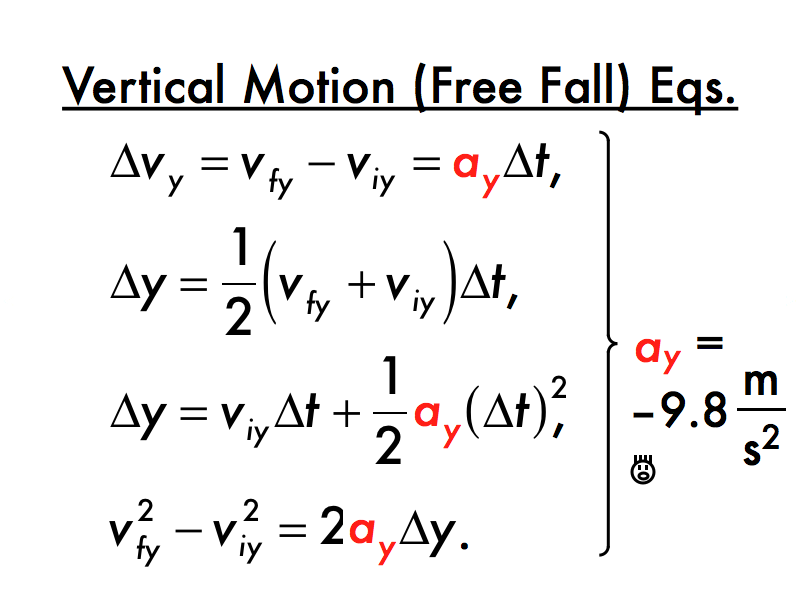
Calculate (i) the maximum height to which it rises, (ii) the total time it takes to return to the surface of the earth.Ī stone is released from the top of a tower of height 19.6 m.ļalculate its final velocity just before touching the ground.Ī stone is thrown vertically upward with an initial velocity of 40 m/s. Calculate (i) the velocity with which the object was thrown upwards & (ii) the time taken by the object to reach the highest pointĪ ball is thrown vertically upwards with a velocity of 49 m/s. Let g = 10 m s–2 (for simplifying the calculations). (i) What is its speed on striking the ground? (ii) What is its average speed during the 0.5 s? (iii) How high is the ledge from the ground?Īn object is thrown vertically upwards and rises to a height of 10 m. A car falls off a ledge and drops to the ground in 0.5 s.


 0 kommentar(er)
0 kommentar(er)
